Indexology^® Blog

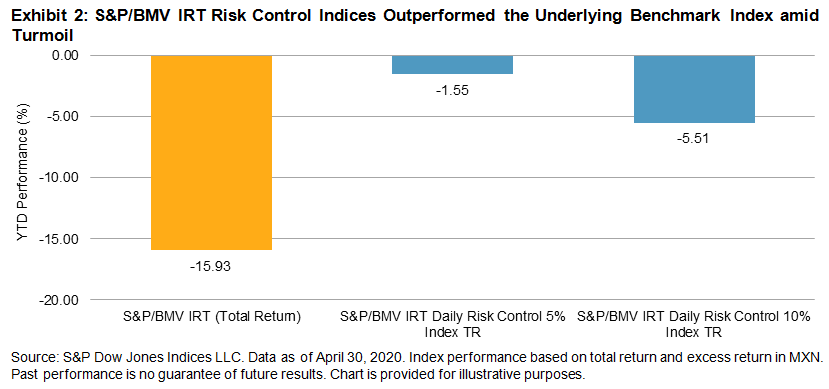
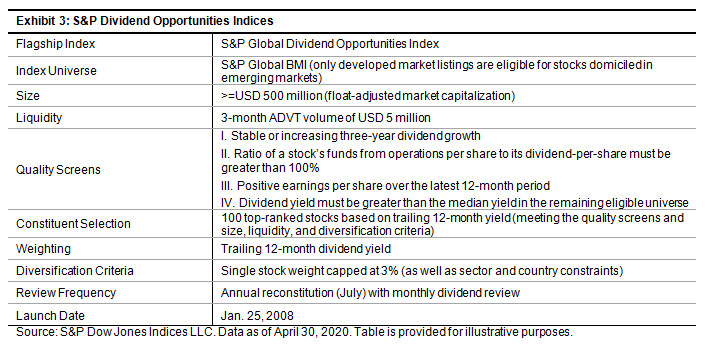
There is a subtle but important distinction between factors and factor indices.  “Factor” denotes an attribute with which long-term excess returns are thought to be associated.  Fama and French, for instance, famously found that small size and cheap valuation were factors in this sense.  A number of other variables – prominently including momentum, low volatility, and quality – have likewise joined the factor pantheon.

Factor indices are designed to provide exposure to one or more of these underlying factors; although quite distinct in their portfolio construction techniques, their commonality stems from the use of factor attributes as a basis for stock selection.  The frequency with which factor indices are rebalanced will influence the degree to which they provide exposure to their target factors.

For example, imagine an index which aims to deliver exposure to the value factor, and so tracks the 50 cheapest stocks in the S&P 500.  Suppose that, over the six months subsequent to its rebalance, most of those stocks become more expensive than the other 450 S&P 500 stocks.  Then our hypothetical value index, which may have perfectly embodied the value factor six months ago, now will embody it less.  The general principle is clear: a factor index best represents its underlying factors at the moment it is rebalanced.  After that, factor drift is not only possible, but likely.

How much drift, and how likely it is, depends on at least two things.  The first of these is the degree to which the factor is observable.  Factors like momentum and low volatility, e.g., depend only on the movement of prices; it’s easy to know whether a stock has high or low momentum.  Factors like quality or growth, on the other hand, are attributes of a company, not a stock price.  They can only be inferred by using accounting or other non-price inputs (which themselves can be observed only at annual or quarterly intervals, and then sometimes with a substantial lag).  To the degree that we anchor our factor definitions in non-price data, we’re likely to observe relatively slower drift.

A second determinant of drift is the degree to which prices change between rebalances.  Here we can base our expectations of drift on the dispersion of stock returns.  Dispersion measures the extent of idiosyncratic price changes within a parent index.  In a period of relatively low dispersion, the prices of most stocks will have changed by a relatively small amount, leading to relatively low potential factor drift.  When dispersion is high, the opposite is true – there will have been a great deal of price movement, and potentially a great deal of factor drift.

Given the spike upward in dispersion that we’ve observed this year, it is likely that turnover for factor indices as they’re rebalanced throughout 2020 will be substantially higher than average.

Investment in Latin America’s equity markets is not for the faint of heart—now more than ever. In the first quarter of 2020, all markets in the region were significantly negative, plagued by recent events. While April has swung markets back to positive territory, we are still far from the proverbial “light at the end of the tunnel.”

In the midst of the continued global pandemic, many countries have started to ease restrictions, as presumed cases of COVID-19 and mortality rates have lowered. This has led to talks of “reopening” economies, infusing investors with courage and optimism—even if it is short lived. With the highest number of recorded COVID-19 infections and deaths globally, the U.S. will look to May 2020 with cautious hope. The million dollar question is when it will be safe for consumers to return to stores and restaurants and to resume travel. Even after businesses and services are fully reopened, many may not have the financial means to participate. In fact, there are already reports of increased debt, credit defaults, and bankruptcies at the business and personal level.

S&P Global Ratings recently reported that credit conditions in emerging markets were worsening. In the latest report,[1] 2020 GDP growth forecasts for Latin America were substantially revised downward. Latin America GDP is expected to fall about 5% in 2020 and a recession is forecast across the region. Economists predict that countries like Chile, which quickly implemented social-distancing policies and offered strong economic stimulus, could have a robust recovery and stand out among its peers. Meanwhile, Mexico and other countries, which delayed public health policies and offered limited stimulus programs, may see weak recoveries. Brazil will most likely be somewhere in the middle.

For now, let’s bask in the latest positive monthly returns for the region. April brought a ray of sunshine to equity indices, as promising treatments and development of vaccines to combat COVID-19 are on the horizon. The S&P 500^® was up 13% for the month, while the S&P Latin American BMI gained nearly 7%. The S&P Latin America 40, which measures the largest, most liquid stocks in the region, gained 5.2%. Among the larger countries, Chile’s S&P IPSA gained 14%, while the S&P Brazil LargeMidCap returned 10%, and Mexico’s S&P/BMV IPC gained nearly 6%. However, none of the country indices gained as much as Argentina’s S&P MERVAL Index, which returned a whopping 34.3%. Despite April’s strong performance, most indices were still in the red YTD. Given all the uncertainty in the markets, continued high volatility is still expected for the rest of the year.

Despite the pandemonium, some indices are standing out from the pack, highlighting how their methodologies function under extreme market conditions. In Mexico, the S&P/BMV IPC Inverse Daily Index, which provides the inverse performance of the S&P/BMV IPC on a daily basis, was up 15.5% YTD. The S&P/BMV IRT Risk Control Indices, which reduce their exposure to equity when volatility rises above pre-defined thresholds (i.e., 5% or 10%, annualized) have outperformed the benchmark.

The S&P/BMV Mexico Target Risk Indices were also winners, thanks to their partial inclusion of fixed income exposure. The series comprises four indices that combine varied levels of equity and fixed income, ranging from conservative to aggressive allocations, while adhering to the constraints of the investment regime of the local pension funds.

The S&P/BMV Ingenius Index seeks to track some of the world’s largest, most innovative, tech companies, which have generally proven to fare well during this pandemic, and seemed to capture this trend YTD. The S&P/BMV China SX20 Index, which is designed to measure separate international stocks trading on Mexico’s BMV, continued to do well largely due to the recent depreciation of the Mexican peso relative to the U.S. dollar. The S&P/BMV MXN-USD Currency Index, which aims to track the change between the currencies, also performed well YTD (see Exhibit 4).

[1]   Tatiana Lysenk, and Elijah Oliveros. “Macroeconomic Developments and Assumptions” in Credit Conditions Emerging Market: Longer Lockdowns, Heightened Risk. S&P Global Ratings, April 23, 2020.

Over the last few months, the COVID-19 pandemic has taken its toll on the global economy in a way that no one could have expected. Unsurprisingly, many companies have had to reassess their dividend policy—in many cases resorting to cutting, postponing, or even suspending dividend payments.

Against this background, it is beneficial to review the selection criteria of S&P DJI’s dividend indices. Not only do all of our dividend indices select constituents based on yield, but they also use a quality screen that focuses on their ability to pay dividends in the future. The quality screen varies with each index and is also dependent on the region.

Simply because a company pays a high dividend (whether absolute amount or relative to its price) doesn’t always make it a wise investment. After all, a company paying too much in dividends may be left with little to no reserves in times of market turbulence; as a result, its dividends may be at higher risk for cancellation. Also, a high dividend yield may simply be a result of a decline in the company’s stock price, all else equal. These scenarios are often referred to as being “dividend traps,” and in order to avoid these traps, it is important to incorporate quality screens.

This blog looks at three core dividend series offered by S&P DJI—S&P Dividend Aristocrats®, Dow Jones Select Dividend, and S&P Dividend Opportunities—with respect to their individual screening features to shed more light on the factors that each considers when selecting constituents.

S&P Dividend Aristocrats Indices

The S&P Dividend Aristocrats Indices are well known for their stringent quality screen which is the hallmark of their methodology. This family focuses on stable dividend growth, requiring constituents to have consistently increased dividends every year for a certain period of time that varies by region.

The longest of these is the S&P 500^® Dividend Aristocrats, for which constituents must have consistently increased dividends every year for at least 25 years, subject to stock diversification criteria (see Exhibit 1).

Dow Jones Select Dividend Indices

The Dow Jones Select Dividend Indices strive to strike a balance between high yield and dividend sustainability. This index series incorporates stability and quality criteria, which are put in place to avoid dividend traps.

The Dow Jones U.S. Select Dividend Index is designed to measure the performance of 100 high-dividend-paying companies that meet specific criteria, excluding REITs. Exhibit 2 includes more details on the index methodology. The quality screens serve to include companies with a solid track record of dividend payments (I and II), sufficient coverage to sustain its current dividend level (III), and positive earnings (IV).

S&P Dividend Opportunities Indices

The S&P Dividend Opportunities Indices are designed to measure the performance of high-yield common stocks from global markets that meet diversification, stability, and tradability requirements.

The S&P Global Dividend Opportunities Index seeks to track 100 common stocks from around the world that offer high dividend yields. As Exhibit 3 shows, constituents are subject to quality screens in order to include companies with a track record of stable or increasing dividend growth (I), enough cash flow from core operations to support dividends (II), and positive earnings (III).

The recent market volatility underscores the importance of including additional criteria, beyond yield, to select constituents within dividend indices. As illustrated above, the different dividend indices offered by S&P DJI attempt to avoid potential pitfalls of dividend investing by incorporating quality screens.

S&P DJI’s Mona Naqvi discusses how the S&P 500 ESG Index could help investors maintain their ESG values without sacrificing performance.

Learn more here: https://www.indexologyblog.com/2020/05/06/whos-in-whos-out-walmart-twitter-dropped-from-the-sp-500-esg-index-and-other-major-changes/

Dividends play a vital role in many investors’ approach to the market, although there is more than one way to approach dividends. Some investors are most concerned with dividend yield per se, while others are more sensitive to the growth of dividends over time.  Both approaches, of course, can be readily indicized.  Within the U.S. large capitalization universe, the S&P 500 High Dividend Index comprises the highest-yielding companies in the S&P 500.  The S&P 500 Dividend Aristocrats, in contrast, consists of S&P 500 members that have increased their dividends annually for at least 25 years.   Exhibit 1 shows that both strategies have outperformed the S&P 500 over the long term.

In the short run, however, investor attention has turned increasingly to the sustainability of dividends, as more companies continue to cut or suspend their payouts. We previously discussed the tradeoff between the level versus the safety of dividend payments, and illustrated the durability of the S&P High Yield Dividend Aristocrats compared to an equivalent universe of high-payers.  Here we conduct a similar analysis for large-cap Aristocrats, comparing them to the S&P 500 High Dividend Index, and see similar results: The S&P 500 Dividend Aristocrats’ dividends are safer than their high yielding peers.

Exhibit 2 compares the median values of the 500 Dividend Aristocrats and the High Dividend Index on a number of fundamental metrics that measure the strength of companies making dividend payouts.

The S&P 500 Dividend Aristocrats appear stronger across the board. For example:

• Earnings were almost double last year’s dividend payouts for the Aristocrats, vs. only 1x dividends for the high payers. Cash on hand was also a higher multiple of last year’s dividends.
• The Aristocrats’ buybacks were more than double those of the high payers, giving them more flexibility during a downturn like the present circumstances.
• Within large-caps, the Aristocrats are 2 times larger (median capitalization $29 billion) and more profitable (median ROE 18.9%) than their higher-paying counterparts.

We again caution that while we do not know how many more companies will cut or suspend their dividends or how severe the impact will be, we can conclude that the dividends of large-cap companies with consistent dividend growth are in healthier shape to withstand economic and market declines.